Compounding is a crucial concept in finance, referring to the process of reinvesting an asset's earnings to generate additional returns over time. This phenomenon, also known as compound interest, is integral to investment portfolios, as it allows for exponential growth in investment value. The frequency of compounding, such as annually, monthly, or daily, plays a significant role in determining the overall returns.
The impact of compounding is best understood through the comparison of simple and compound interest. While simple interest calculates returns solely based on the principal amount, compound interest includes reinvested earnings, leading to interest on interest and accelerated investment growth.
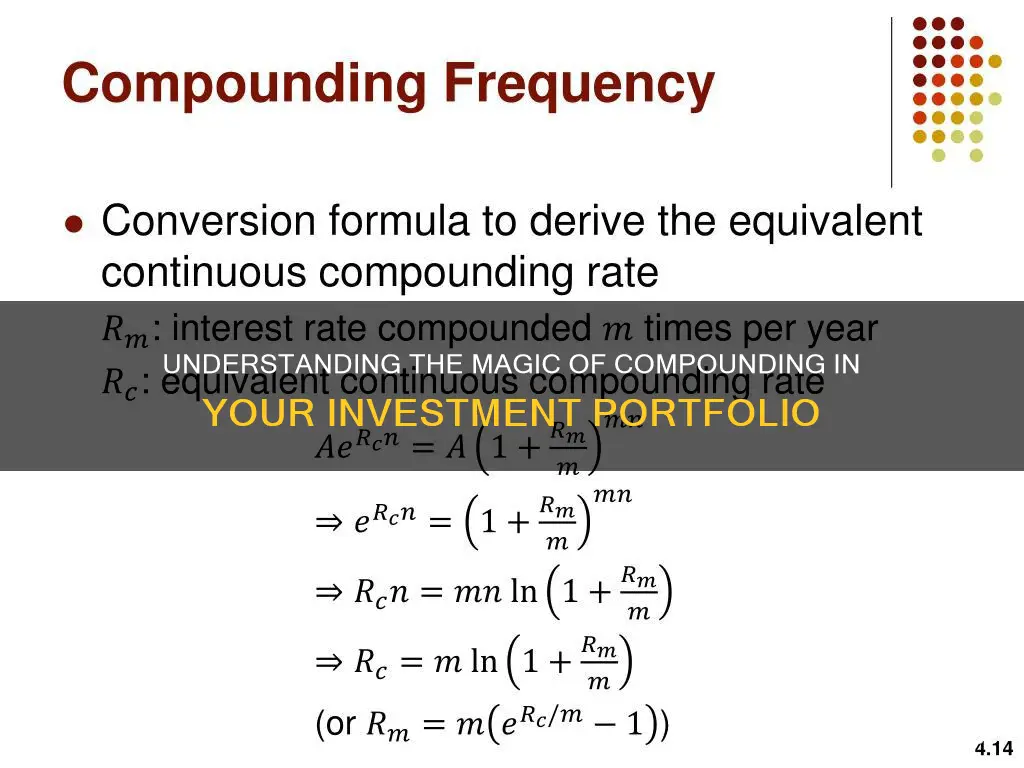
The choice of compounding frequency depends on the expected return and the time frame. Although yearly, monthly, or daily compounding intervals are common, more frequent compounding generally leads to faster investment growth.
Understanding the concept of compounding is essential for investors to maximize their investment returns and build wealth over time.
| Characteristics | Values |
|---|---|
| How often is an investment portfolio compounded? | Compounding can occur weekly, monthly, quarterly, biannually, or annually. |
| Effect of compounding frequency | More frequent compounding leads to faster growth. |
| Compounding and risk | Compounding can work for or against an investor depending on their financial situation. |
| Compounding and debt | Compound interest can increase the amount of money owed on a loan as interest accumulates on the unpaid principal and previous interest charges. |
What You'll Learn

Compounding frequency and its impact on investment growth
Compounding frequency refers to the number of times the interest is added to the principal in a given period. The higher the number of compounding periods, the greater the compound interest growth will be. The compounding frequency can be annual, semi-annual, quarterly, monthly, weekly, or daily. Some banks also offer continuously compounding interest, which adds interest to the principal as regularly as possible.
The impact of compounding frequency on investment growth is significant. The more frequent the compounding, the faster the investment growth. For example, $10,000 invested at 8% interest compounded annually would result in a lower balance than if compounded quarterly or monthly. This is because, with more frequent compounding, the interest is added to the principal more often, leading to higher overall returns.
In the case of stocks, the impact of compounding frequency is less straightforward. While more frequent compounding can still boost returns, other factors come into play, such as fees and the number of shares purchased. Additionally, stocks may not follow a predictable compounding curve due to fluctuations in share prices and dividend payouts.
It is important to note that the choice of compounding frequency may not make a significant difference for low-interest rates or short time frames. However, for higher interest rates and longer periods, the compounding frequency can have a noticeable impact on the estimated returns.
Savings and Investments: Equation for Financial Success
You may want to see also

Compounding intervals and their effect on returns
Compounding intervals refer to the frequency with which interest is added to the principal amount of an investment or loan. The compounding frequency can have a significant effect on the overall returns of an investment or the total amount of debt owed.
The compounding frequency can vary, and common intervals include annually, semi-annually, quarterly, monthly, weekly, and daily. Some investments or loans may also offer continuous compounding, where interest is added as regularly as possible, approaching an infinite number of compounding periods per year.
The impact of compounding intervals is that more frequent compounding leads to faster growth or accumulation of debt. For example, consider an investment of $1,000 at a 5% interest rate. With simple interest, the investor would earn $50 every year. However, with compound interest, the interest is earned on the growing principal amount. In the first year, the investor earns $50, and in the second year, the interest is calculated on $1,050, resulting in $52.50 of interest. This process continues, and the investor's returns grow over time.
The effect of compounding intervals becomes more pronounced over longer periods of time. For instance, $10,000 invested at an 8% interest rate for 30 years would grow to $115,865 with annual compounding. However, with quarterly compounding, the final amount would be $121,550, and with daily compounding, it would be $122,134.
It is important to note that the choice of compounding frequency may not make a significant difference for short-term investments or low-interest rates. Nonetheless, for long-term investments or higher interest rates, the compounding interval can have a notable impact on the overall returns.
Savings and Investment: National Strategies for Economic Growth
You may want to see also

How compound interest differs from simple interest
When it comes to calculating interest, there are two basic choices: simple interest and compound interest. This is how they differ:
Simple Interest
Simple interest is calculated on the principal, or original, amount of a loan. It is charged only on the original principal amount. It is calculated by multiplying the loan principal by the interest rate and then by the term of the loan. For example, if you invest $1,000 at 5% simple interest for 10 years, you can expect to receive $50 in interest every year for the next decade. No more, no less.
Compound Interest
Compound interest is calculated on the principal amount and the accumulated interest of previous periods. It is often referred to as "interest on interest". It multiplies savings or debt at an accelerated rate. Compound interest is calculated on both the initial principal and all of the previously accumulated interest.
For example, if you invest $1,000 at 5% interest for the first year, you receive a $50 interest payment. If you reinvest that at the same rate, your second-year interest will be calculated on a $1,050 investment, which comes to $52.50. In the third year, your interest will be calculated on $1,102.50, and so on.
How They Differ
The difference between simple and compound interest can be massive. Take a $10,000 investment portfolio at 10% interest over time. With simple interest, you will receive a flat $1,000 every year. With compound interest, that same investment will grow to $174,494 in 30 years.
Compound interest can be a potent factor in wealth creation. It can help you grow your savings and reach your financial goals. It is also an easy way to boost your earnings without taking on any additional risk.
However, compound interest can work against you if you have debt. Credit card companies often utilise compound interest to charge consumers more money. If you carry a balance on your credit card, the interest you're charged will be compounded, leading to an even higher balance.
Diversification: Managing Risk in Your Investment Portfolio
You may want to see also

The Rule of 72 for estimating doubling time
The Rule of 72 is a quick and useful formula for estimating the number of years required for an investment to double based on a given annual rate of return. It can also be used to calculate the annual rate of compounded return on an investment given the number of years it will take to double.
The Rule of 72 is a simplified formula that calculates how long it will take for an investment to double in value based on its rate of return. It is calculated by dividing 72 by the expected rate of return. For example, if an investment scheme promises an 8% annual compounded rate of return, it will take approximately nine years (72 / 8 = 9) to double the money invested.
The Rule of 72 is a handy tool for mental calculations to quickly gauge an approximate value. It is often taught to beginner investors as it is easy to understand and calculate. The Security and Exchange Commission also cites the Rule of 72 in grade-level financial literacy resources.
The Rule of 72 is reasonably accurate for interest rates that fall in the range of 6% to 10%. For rates outside this range, the rule can be adjusted by adding or subtracting 1 from 72 for every 3 points the interest rate diverges from the 8% threshold. For instance, for a 5% rate of return, the rule would be adjusted to 71 (72 - 1 = 71) for greater precision.
The Rule of 72 can also be used to estimate the impact of fees on investment policies, such as mutual fund fees and insurance investment portfolio charges. By dividing 72 by the fee, you can estimate the number of years it will take for the total account value to halve.
Additionally, the Rule of 72 can be applied to anything that grows at a compounded rate, such as population, macroeconomic numbers, charges, or loans. For example, if the gross domestic product (GDP) grows at 4% annually, the economy is expected to double in 18 years (72 / 4% = 18 years).
The Rule of 72 is a simplified version of the more complex compound interest calculation. It is a useful rule of thumb for estimating the doubling of an investment.
Savings-Investment Equilibrium: What's the Balancing Act?
You may want to see also

How to calculate compound interest
The concept of compounding is an important one in investing, allowing you to grow your money over time. Compounding can be applied to both investments and loans, although the latter is beyond the scope of this discussion.
How Compounding Works
Compounding is the process of reinvesting earnings, thereby increasing the principal and generating larger and larger interest payments over time. This is also known as "interest on interest".
For example, if you invest $1,000 at a 5% interest rate, you will receive $50 in interest at the end of the first year. If you reinvest this $50 at the same 5% rate, you will earn $52.50 in interest at the end of the second year, as the interest is calculated on the new principal of $1,050.
The compound interest formula is as follows:
> A = P(1 + r/n)nt - P
- A = Final amount (principal + interest)
- P = Principal starting amount
- R = Annual nominal interest rate as a decimal
- N = Number of compounding periods per unit of time
- T = Time in years
If the interest is compounded annually, you would simply add the interest to the principal once per year. For semi-annual compounding, you would apply half the interest rate twice a year. Other common compounding frequencies include quarterly, monthly, weekly, and daily.
Let's say you invest $20,000 at an interest rate of 5%, compounded quarterly, for 20 years.
- P = $20,000
- R = 0.05 (5% expressed as a decimal)
- N = 4 (compounded quarterly, i.e. four times a year)
- T = 20 years
Plugging these values into the formula, we get:
> A = $20,000(1 + 0.05/4)4 x 20 - $20,000
Calculating this gives us the final amount, A, of $72,384.75.
Factors Affecting Compound Interest
The frequency of compounding can have a significant impact on the growth of your investment. More frequent compounding intervals will accelerate investment growth. For example, $10,000 invested at an 8% interest rate compounded annually would grow to $10,640 after 5 years. However, if the interest is compounded quarterly, the final amount would be $10,651.11, and if compounded monthly, it would be $10,652.27.
Additionally, the length of time your money is invested can also make a big difference. The longer you keep your money invested, the more time it has to grow, and the larger the impact of compounding.
It is also worth noting that compound interest is always greater than or equal to simple interest, where the interest is calculated only on the principal amount.
Building an Investment Portfolio: A College Graduate's Guide
You may want to see also
Frequently asked questions
There is no concept of compounding for investments in individual stocks. However, compounding can occur if dividends are reinvested.
Simple interest is a set percentage of the principal amount every year. Compound interest is when interest is earned on both the principal and the interest that's added to the balance over time.
More frequent compounding intervals can accelerate investment growth and enhance overall returns.