A minimum variance portfolio is a fancy way of saying a low-risk portfolio. It carries low volatility as it correlates to your expected return, meaning you're not taking on more risk than is necessary. It consists of individually risky assets that are hedged when traded together, resulting in the lowest possible risk for the rate of expected return.
The minimum variance portfolio is based on the Markowitz Portfolio Theory, which suggests that volatility can be used to replace risk and, therefore, less volatility variance correlates with less investment risk.
This type of portfolio is useful for investors who want to reduce their risk during market sell-offs and preserve value during extreme sell-offs, so their portfolios are well-positioned to gain value when markets recover.
| Characteristics | Values |
|---|---|
| Definition | A well-diversified portfolio that consists of individually risky assets, which are hedged when traded together, resulting in the lowest possible risk for the rate of expected return |
| Origin | The term originates from the Markowitz Portfolio Theory |
| Volatility | Low volatility as it correlates to your expected return |
| Correlation | Holds individual, volatile securities that aren't correlated with one another |
| Risk | A low-risk portfolio |
| Risk Measurement | Variance is a measurement of risk |
| Diversification | Achieve higher returns without exposing you to more risk |
| Risk-Return Relationship | Minimum variance (minimum risk) portfolios have produced greater returns than higher-risk portfolios |
| Risk Metric | Expected shortfall (ES) is the best risk metric |
What You'll Learn

Understanding the basics of a minimum variance portfolio
A minimum variance portfolio (MVP) is a well-diversified portfolio that consists of individually risky assets. When traded together, these assets are hedged, resulting in the lowest possible risk for the expected rate of return. In other words, it is a fancy way of saying a low-risk portfolio.
The term originates from the Markowitz Portfolio Theory, which suggests that volatility can be used to replace risk, meaning less volatility variance correlates with less investment risk. This theory was developed by Harry Markowitz, who won a Nobel Prize for his work in the 1950s.
The key to an MVP is to hold investments with a low correlation to each other. For example, one security might be surging in value while another is plummeting, but because of their low correlation, the portfolio as a whole is viewed as less risky. Any two investments with a low correlation to each other can form an MVP, such as stocks and bonds.
Variance is a measurement of risk and measures the daily fluctuations of an investment. The more significant the change in price, the higher its variance, and the more volatile it is. The standard deviation of an investment is the square root of the variance, and both measure portfolio risk.
Correlation measures the movements between investments. If two assets are negatively correlated, they will move in opposite directions. When implementing an MVP, you should look for investments with low or negative correlations. You should also aim for diversification across asset classes.
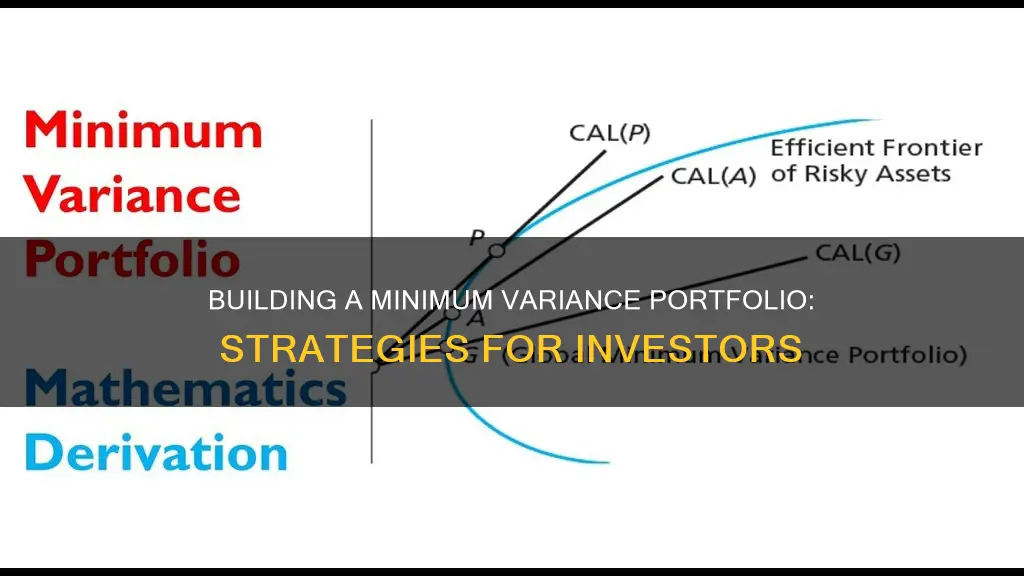
The Efficient Frontier is a graph that rates your portfolio's risk (x-axis) versus return (y-axis). It shows the amount of profit you can expect from an assumed level of risk. It helps you determine if you are taking too much risk for the reward you are hoping for and if you need to diversify by adding more investments to your portfolio.
While constructing an MVP can be complicated, the basic principle is to keep it simple. Invest in basic asset classes such as stocks, bonds, and cash. This will still give you an MVP, just a simpler one.
Accessing Your ENT Investment Portfolio: A Step-by-Step Guide
You may want to see also

The importance of diversification
At its core, diversification involves spreading investments across a variety of assets and asset classes, rather than concentrating them in a single asset or class. By doing so, investors can lower the impact of fluctuations in any one investment or class on their overall portfolio performance. This is often summed up by the proverb, "Don't put all your eggs in one basket."
In practical terms, a well-diversified portfolio might include a mix of stocks, bonds, cash alternatives, real estate, and commodities. Each of these asset classes carries different levels of risk and potential returns, and they tend to perform differently under similar market conditions. For example, stocks are generally the riskiest but offer the greatest growth potential, while bonds are less volatile but provide more modest returns.
Diversification also extends to investing in assets with low or negative correlations. This means that if one investment is surging, another might be falling, but due to their low correlation, the overall portfolio risk is reduced. For instance, stocks and bonds often exhibit negative correlation, so in the event of a stock market correction, bonds can provide balance and potentially offset any losses.
The benefits of diversification are significant. Firstly, it helps to reduce the risk of not meeting future financial goals by minimising the impact of poor-performing assets. Secondly, it allows investors to better withstand dips in performance and stay the course towards their financial objectives. Finally, diversification can reduce the pain of losses, as the psychological impact of a loss is twice as powerful as the pleasure of a gain, according to behavioural scientists.
In conclusion, diversification is vital when constructing a minimum variance portfolio. By spreading investments across asset classes, investors can lower risk, smooth out performance fluctuations, and increase the likelihood of achieving their financial goals.
Invest Wisely to Secure Your Dream Home
You may want to see also

How to calculate portfolio risk
Calculating portfolio risk is an important step in constructing a minimum variance portfolio. Here's a step-by-step guide on how to calculate portfolio risk:
Understand Portfolio Variance:
Portfolio variance is a statistical measure of the volatility or risk associated with a portfolio of investments. It takes into account the individual variances of each asset in the portfolio, as well as their co-variances or correlation coefficients. By calculating the portfolio variance, you can quantify the overall risk of the portfolio.
Identify the Components of Portfolio Variance:
The formula for portfolio variance in a two-asset portfolio is:
Portfolio Variance = w1²σ1² + w2²σ2² + 2w1w2Cov1,2
- W1 and w2 represent the portfolio weights of the first and second assets, respectively.
- Σ1 and σ2 represent the standard deviations of the first and second assets, respectively.
- Cov1,2 is the co-variance of the two assets, which can be expressed as the correlation coefficient between the two assets multiplied by their standard deviations: p(1,2)σ1σ2.
Collect Data on Individual Assets:
To calculate portfolio variance, you need to gather data on each asset in the portfolio. This includes the weight or proportion of each asset in the portfolio, the standard deviation of each asset's returns, and the co-variance or correlation coefficient between each pair of assets.
Calculate the Portfolio Variance:
Plug the values you've collected into the formula. Multiply the squared weight of each asset by its corresponding variance, and then add twice the product of the weighted average weight and the co-variance of all individual security pairs. This will give you the portfolio variance.
Interpret the Results:
A lower portfolio variance indicates a lower risk associated with the portfolio. By comparing portfolio variances for different combinations of assets, you can identify portfolios with lower risk profiles.
Consider Modern Portfolio Theory (MPT):
MPT suggests that investors seek to maximise returns while minimising risk. The efficient frontier, a graph with risk on the x-axis and return on the y-axis, helps visualise this relationship. Portfolios that fall on the efficient frontier offer the best return for a given level of risk.
Apply the Minimum Variance Approach:
The minimum variance approach, influenced by Harry Markowitz's MPT, suggests selecting assets with negative or low correlation to minimise portfolio variance. This means choosing assets that don't move in the same direction, reducing the overall risk of the portfolio.
Diversify Your Portfolio:
Diversification is a key aspect of the minimum variance approach. By investing in a variety of sectors, company sizes, and asset classes, you can further reduce unsystematic risk. Diversification ensures that a loss in one particular sector or asset class won't significantly affect the overall portfolio.
Monitor and Adjust:
Regularly review the performance and risk metrics of your portfolio. If the standard deviation or portfolio variance is deemed too high, you can adjust your holdings by incorporating assets with lower correlations to potentially reduce the overall risk.
By following these steps and calculating portfolio risk, you can construct a minimum variance portfolio that aligns with your investment goals and risk tolerance.
Creating Investment Portfolio Projections: A Comprehensive Guide
You may want to see also

The role of the Sharpe Ratio in risk management
The Sharpe Ratio is an important tool for risk management when investing in a minimum variance portfolio. A minimum variance portfolio is a well-diversified portfolio of individually risky assets that are hedged when traded together, resulting in the lowest possible risk for the expected rate of return.
The Sharpe Ratio, proposed by economist William F. Sharpe in 1966, is a way to measure the performance of an investment by adjusting for its risk. It compares the return of an investment with its risk, indicating whether excess returns are due to smart investment decisions or simply luck and risk. The ratio is calculated by subtracting the risk-free rate of return from the expected rate of return and then dividing that result by the standard deviation (a measure of volatility and risk).
The Sharpe Ratio helps investors understand the level of risk they are taking on for the potential return. A higher ratio implies a higher investment return relative to the risk taken. A ratio of 1 or above is considered good, with 2 or above considered very good, and 3 or above considered excellent.
The Sharpe Ratio is useful when evaluating different investment options or comparing the performance of investment managers. For example, when comparing two fund managers, one with a higher return but a lower Sharpe Ratio, it indicates that the manager with the lower return is generating better risk-adjusted returns.
The Sharpe Ratio has some limitations and inherent weaknesses. It assumes that price movements in either direction are equally risky, which may not always be the case. Additionally, the standard deviation calculation used in the ratio may understate tail risk and is most useful for evaluating symmetrical probability distribution curves.
In conclusion, the Sharpe Ratio is a valuable tool for risk management when investing in a minimum variance portfolio. It helps investors understand the return potential relative to the risk taken and make more informed investment decisions. However, it is important to be aware of the ratio's limitations and use it in conjunction with other tools and analyses.
Monthly or Annually: Which Investing Strategy Wins?
You may want to see also

Constructing a minimum variance portfolio
Step 1: Understanding Minimum Variance Portfolios
Before constructing a minimum variance portfolio, it is essential to understand its fundamental characteristics. A minimum variance portfolio is an investment strategy that aims to minimise risk and maximise profits. It is a type of portfolio structuring that focuses on reducing investment risks while maximising profit potential. In a minimum variance portfolio, individual assets with some level of risk are combined so that, when put together, they hedge against each other, resulting in the lowest attainable level of risk for a given set of assets.
Step 2: Identifying Low Volatility Investments
When building a minimum variance portfolio, the investor needs to identify multiple investment opportunities with low volatility. Volatility refers to the price movement of an investment. The goal is to find investments with low or negative return correlations, meaning their prices do not move in the same direction. This can include a mix of stocks, bonds, commodities, and other investment vehicles from various sectors, industries, and geographical regions.
Step 3: Diversification and Low Correlation
Diversification is a critical aspect of constructing a minimum variance portfolio. Investors should look for investments that are not similar and are unlikely to perform the same way, especially in the same economic environment or market. This means including assets from different sectors and industries in the portfolio. For example, bonds and stocks are a classic combination in a minimum variance portfolio because they often move in opposite directions. When stocks are performing well, bond prices tend to be negative or flat, and when stocks are bearish, bond prices turn bullish.
Step 4: Calculating Portfolio Variance
To construct a minimum variance portfolio, it is necessary to calculate the portfolio variance, which requires evaluating the standard deviations of each security within the portfolio and factoring in the correlations between each security pair. The formula for calculating portfolio variance is: Portfolio Variance = Σ Wi^2 * σi^2 + 2 * Σ Wi * Wij * cov(i,j), where Wi is the weight of asset i, σi is the standard deviation of asset i, and cov(i,j) is the covariance of assets i and j.
Step 5: Optimisation Techniques
There are various optimisation techniques used in constructing a minimum variance portfolio, such as mean-variance optimisation and the risk-parity approach. Mean-variance optimisation involves solving a quadratic programming problem to find the optimal asset allocation that minimises the portfolio's variance while considering constraints such as target return or asset weight limits. The risk-parity approach, on the other hand, aims to equalise the contribution of each asset to the overall portfolio risk by allocating more capital to lower-risk assets and less to higher-risk ones.
Step 6: Monitoring and Rebalancing
Rajiv Gandhi Equity Savings Scheme: A Smart Investment Move
You may want to see also
Frequently asked questions
A minimum variance portfolio is a low-risk portfolio that consists of individually risky assets that are hedged when traded together, resulting in the lowest possible risk for the expected rate of return.
A minimum variance portfolio allows you to maximise the benefits of diversification. It helps you to achieve higher returns without exposing you to more risk.
Minimum variance portfolios differ from traditional portfolios in how they allocate assets. Minimum variance portfolios are driven by how assets contribute to total portfolio risk, not by a fixed percentage allocation.
You can construct a minimum variance portfolio by calculating the average weighted risk and total portfolio risk for each asset. You can then select the allocation with the lowest total portfolio risk.
Examples of minimum variance portfolios include the Larry Portfolio and Harry Browne's Permanent Portfolio. The Larry Portfolio allocates 70% of its assets to bonds and 30% to volatile equity funds. The Permanent Portfolio evenly splits its assets between stocks, bonds, gold, and cash.







