Standard deviation is a statistical measure used to assess the volatility of an investment. It is calculated using a series of numbers, with the first step being to find the mean or average. The second step is to determine the range of returns, measured from the mean. Standard deviation is a way to measure the risk of an investment, with a higher standard deviation indicating a riskier investment. It is used to determine the consistency of an investment's return over time, with a low standard deviation indicating more predictable returns. Standard deviation is a commonly used metric in investment analysis to help investors make better decisions.
| Characteristics | Values |
|---|---|
| Used to determine | Market volatility or the spread of asset prices from their average price |
| Used to calculate | The degree to which an individual value in a probability distribution tends to vary from the mean of the distribution |
| Used to assess | The riskiness of an asset |
| Used to predict | Performance trends |
| Used to compare | The volatility of different assets |
| Used to identify | Highly volatile stocks |
| Used to determine | How stable or predictable an investment is likely to be |
What You'll Learn

Standard deviation and volatility
Standard deviation is a statistical measure of volatility, or the average amount by which individual data points differ from the mean. In investment analysis, standard deviation is used to determine the riskiness of an investment. The higher the standard deviation, the higher the volatility and the risk.
When applied to the annual rate of return of an investment, standard deviation provides information on that investment's historical volatility. It shows how much the price of that investment has fluctuated over time. A volatile stock has a high standard deviation, while a stable blue-chip stock usually has a low standard deviation.
Standard deviation is calculated by first working out the mean of all data points. Then, the variance for each data point is calculated by subtracting the mean from the value of the data point. The variance of each data point is then squared, and these squared values are added together and divided by the number of data points in the set. Finally, the square root of this quotient is taken to give the standard deviation.
Standard deviation is one of the most common methods of determining the risk an investment poses. It is used by investment analysts to understand the risks associated with a mutual fund or hedge fund. It is also used by businesses to assess risk, manage operations, and plan cash flows based on seasonal changes and volatility.
Standard deviation is a useful tool for investors to make investment decisions that align with their risk tolerance and financial circumstances. For example, retirees may prefer more reliable returns to fund their retirement, rather than potentially navigating periods where assets return far less than average. However, standard deviation might not be the only risk measure to consider when making investment decisions.
Fidelity Investments: A Guide to Getting Started
You may want to see also

Standard deviation and risk
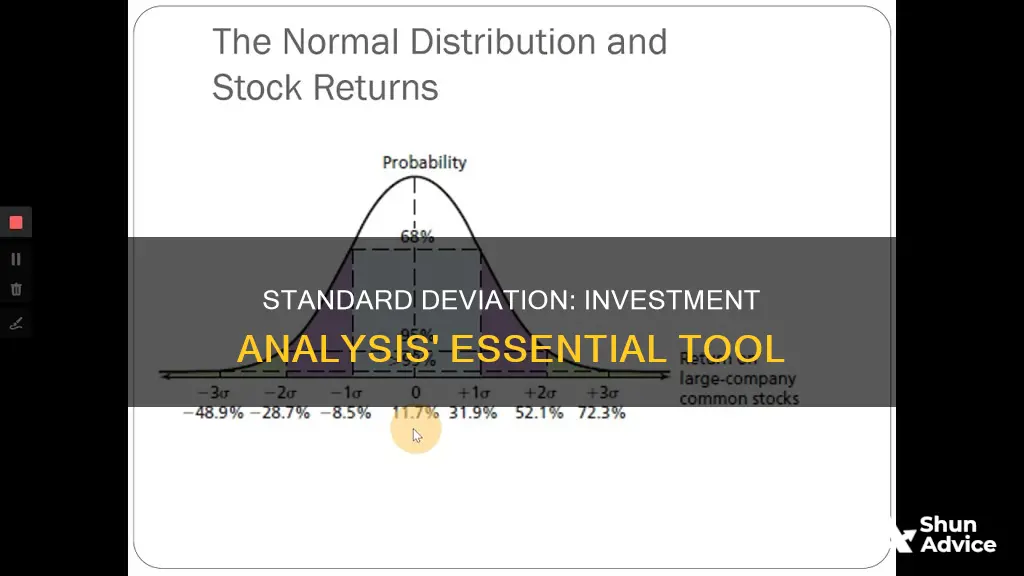
Standard deviation is a statistical measure of volatility, or risk, in investment analysis. It is a commonly used metric for determining the risk an investment poses. Standard deviation measures the degree to which an individual value in a probability distribution tends to vary from the mean, or average, of the distribution. This is often represented as a bell curve.
In the context of investments, standard deviation helps determine market volatility or the spread of asset prices from their average price. It provides a measure of how much an investment's price has fluctuated over time, indicating how stable or predictable an investment is likely to be. A volatile stock has a high standard deviation, while a stable blue-chip stock usually has a low standard deviation.
The standard deviation of an investment's performance can be calculated by first determining the mean or average. The second step is to determine the range of returns, measured from the mean. This range of returns is then used to calculate the standard deviation.
The higher the standard deviation, the higher the risk. A large standard deviation indicates that there is a lot of variance in the observed data around the mean, meaning the data is quite spread out. Conversely, a small or low standard deviation indicates that the data is clustered tightly around the mean, suggesting more consistent and predictable returns.
Standard deviation is an important tool for investment analysts to assess the risks associated with a mutual fund or hedge fund. It helps them understand the consistency of returns over time. A mutual fund with consistent returns will have a low standard deviation, while a growth-oriented or emerging market fund with greater volatility will have a higher standard deviation and be considered riskier.
Standard deviation is also used by businesses to assess risk, manage operations, and plan cash flows based on seasonal changes and volatility. It is a valuable tool for risk management, helping businesses quantify and manage different types of risks.
Investing Cash: How Much and When?
You may want to see also

Standard deviation and consistency
Standard deviation is a statistical measure of volatility, or consistency, in investment analysis. It is a way to quantify the risk of an investment by measuring how far individual data points are from the mean. In other words, it shows how much the returns on an investment deviate from a particular set of historical data. This data can be measured against itself (past prices of the same security) or compared to an average or "benchmark".
The higher the standard deviation, the higher the volatility and risk. Conversely, a lower standard deviation indicates more consistent returns and lower risk. For example, a volatile stock has a high standard deviation, while a stable blue-chip stock usually has a low standard deviation.
Standard deviation is calculated by first finding the mean of a dataset. Then, the difference between the mean and each data point is found and squared. These squared differences are then added together and divided by the number of data points in the set. Finally, the square root of this number gives the standard deviation.
It's important to note that standard deviation assumes a normal distribution of data, which may not always be the case. Additionally, it treats all uncertainty as risk, even when it is in the investor's favour. Despite these limitations, standard deviation is a valuable tool for investors to assess the risk and potential returns of an investment.
MACD for Hourly vs Daily Investing: Which Timeframe Wins?
You may want to see also

Standard deviation and historical patterns
Standard deviation is a statistical measure of volatility, or the degree to which an individual value in a probability distribution tends to vary from the mean. In the context of investment analysis, standard deviation is used to determine the riskiness of an investment. It helps investors understand how consistent or unpredictable an investment's returns are likely to be over time.
When applied to the annual rate of return of an investment, standard deviation provides information on its historical volatility. It shows how much the price of that investment has fluctuated over time. A higher standard deviation indicates greater variance between each price and the mean, resulting in a larger price range. For example, a volatile stock typically has a high standard deviation, with its price frequently going up and down. On the other hand, a stable blue-chip stock usually exhibits a low standard deviation, indicating that its price tends to remain stable.
Standard deviation can also be used to predict performance trends. Index funds, designed to replicate a benchmark index, typically have a low standard deviation from the value of that benchmark. Conversely, aggressive growth funds often exhibit a high standard deviation from relative stock indices due to their portfolio managers' aggressive bets aimed at generating higher-than-average returns.
In investment analysis, standard deviation is a key tool for analysts, portfolio managers, and advisors. It is one of the most common methods for determining the risk posed by an investment. By examining the standard deviation of historical returns, investors can gain insights into the potential volatility and risk associated with a particular investment. This information can then be used to make informed decisions that align with their risk tolerance and financial goals.
It is important to note that standard deviation is based on past data, and future performance may differ. Additionally, standard deviation is just one aspect of investment analysis, and other factors and metrics should also be considered when evaluating investment opportunities.
Understanding Cash Investments in Your Portfolio
You may want to see also

Standard deviation and future predictions
Standard deviation is a statistical measurement that calculates the volatility of an investment. It is the most frequently used metric for determining the risk an investment poses. By measuring the volatility of an investment, standard deviation can be used to predict future performance trends.
Standard deviation calculates the degree to which individual values in a probability distribution tend to vary from the mean. In the context of investments, it is calculated using monthly returns over a historical period, typically 36 months. This calculation results in a single number that represents an asset's performance swings over that period.
The higher the standard deviation, the higher the volatility and risk of an investment. A volatile stock has a high standard deviation, while a stable blue-chip stock usually has a low standard deviation. For example, an index fund designed to replicate a benchmark index will have a low standard deviation from the value of the benchmark. On the other hand, aggressive growth funds often have a high standard deviation due to aggressive bets made by portfolio managers.
Standard deviation is also used to predict future performance trends. In investing, standard deviation can indicate how often the price of a stock or other financial instrument is likely to deviate from its average value. For instance, a share of a company's stock trading at $10 per share, with a standard deviation of two, indicates that the value of the stock will likely be between $8 and $12 per share 68% of the time.
It is important to note that standard deviation is based on historical data and may not be indicative of future performance. Additionally, standard deviation assumes a normal distribution of data, which may not always be the case for investment portfolios.
By understanding the standard deviation of an investment, investors can evaluate the potential volatility and rate of return. A higher standard deviation indicates greater volatility and the potential for larger price swings, while a lower standard deviation suggests more predictable returns and lower risk.
Cash Value Annuities: Securities Investment Options Explored
You may want to see also
Frequently asked questions
Standard deviation is a statistical measurement that calculates the degree to which an individual value in a probability distribution tends to vary from the mean of the distribution. It is often used as a measure of volatility and risk in investment analysis.
Standard deviation is calculated by first determining the mean or average of a dataset. Then, the range of returns is measured by finding the difference between each data point and the mean. These differences are squared, added together, and divided by the number of data points minus one. Finally, the square root of this result is taken to obtain the standard deviation.
Standard deviation is used in investment analysis to assess the risk and volatility of an investment. A higher standard deviation indicates that an investment is more volatile and riskier, while a lower standard deviation suggests that the investment is more stable and predictable.
Standard deviation has some limitations. It assumes a bell-shaped distribution of data, which may not be accurate for all portfolios, especially hedge funds. Additionally, standard deviation is based on past data, which may not be indicative of future performance. It also does not measure how far a data point is from the mean directly but instead compares the square of the differences.







