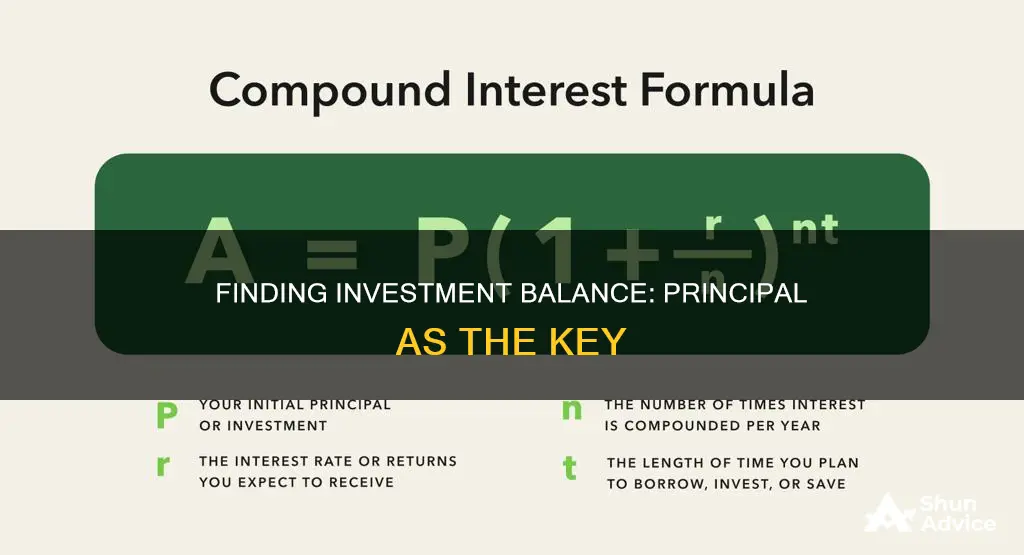
The principal amount is the original sum of money put into an investment or borrowed in a loan. It is the foundation for calculating interest on a loan or the returns on an investment. When it comes to loans, the principal amount owed changes over time as it is paid off. This is why there are two types of principal balances: the initial principal and the outstanding principal. The initial principal is the original amount borrowed, while the outstanding principal refers to the amount still owed on a loan after payments have been made. To calculate the outstanding principal, you can use a loan calculator or a formula that takes into account the interest rate, the number of payments made, and the remaining balance.
| Characteristics | Values |
|---|---|
| Principal amount definition | The original sum of money that’s borrowed in a loan or placed into an investment |
| Principal in loans | The initial amount borrowed from the lender |
| Principal in investments | The original amount of money invested |
| Principal in bonds | The face value of a bond that will be returned to the bondholder when it matures |
| Principal calculation formula | P = I/rt |
| Simple interest formula | I = Prt |
| Simple interest plus principal formula | A = P(1 + rt) |
| Compound interest | Interest that accumulates is added back into the balance of the loan or investment principal |
What You'll Learn

How to calculate the principal amount
The principal amount is the original sum of money put into an investment or borrowed in a loan. It is also the face value of a bond or security. The definition of the principal amount varies based on its application.
To calculate the principal amount of a loan, you can use the following formula:
Principal Amount = EMI (Equated Monthly Instalment) - Interest Amount
Alternatively, the formula for calculating the principal amount is:
Principal Amount (P) = Interest Amount (I) / (Interest Rate (R) x Number of Years (T))
For example, if you deposit $70,000 into a savings account with an interest rate of 5% per year, after seven years, your total amount will be $75,000. The initial invested amount of $70,000 is the principal, and the remaining $5,000 is the interest earned.
In the case of loans, the principal amount refers to the actual amount borrowed from the lender and the remaining amount after partial repayment. For instance, if you take out a home loan of $250,000 and have already repaid $100,000, the initial principal amount was $250,000, but it is now $150,000.
When calculating the principal and interest on a simple interest loan, you can multiply the principal by the interest rate and then multiply the result by the loan term. For instance, if you borrow $100,000 at a simple interest rate of 5% per year for two years, the interest for each year would be $5,000, resulting in a total repayment of $110,000.
The calculation of principal and interest becomes more complex for loans that use other interest calculations, such as amortised loans (e.g., mortgages) or compound interest (e.g., credit cards). In these cases, a loan calculator can be helpful in determining the interest payments.
Unveiling Annual Investments: BA II Plus Calculator Magic
You may want to see also

How to calculate interest and principal in an EMI
When you take out a loan, you will have to pay it back over a set period of time, as agreed with the bank or financial institution. This repayment will include both the loan amount and the interest charged on it. This total amount is then divided into equal monthly payments, known as Equated Monthly Instalments (EMI).
The EMI comprises the interest on the loan and part of the principal amount to be repaid. The exact percentage of the EMI that goes towards the interest and principal will change over time, with the interest component being larger in the initial months and then gradually reducing.
There are several ways to calculate the interest and principal components of an EMI. One way is to use an online EMI calculator, which will require details such as the principal loan amount, interest rate, and loan term. Another way is to use a mathematical formula, which can be done manually or with the help of tools like Excel.
EMI = P x R x (1+R)^N / [(1+R)^N-1]
- P stands for the principal loan amount.
- N is the loan tenure in months.
- R is the monthly interest rate, calculated as: R = Annual Rate of Interest/12/100.
For example, if you borrow ₹1,000,000 at an annual interest rate of 10.5% for a period of 10 years (120 months), the EMI would be calculated as follows:
EMI = ₹1,000,000 x 0.00875 x (1 + 0.00875)^120 / ((1 + 0.00875)^120 - 1) = ₹13,493.
So, you would need to pay ₹13,493 every month for 120 months to repay the entire loan amount. The total amount payable would be ₹13,493 x 120 = ₹16,19,220, which includes ₹6,19,220 as interest.
To calculate the interest and principal components of a particular month's EMI, you can use the following formulas:
- Interest component = P x R
- P stands for the remaining principal loan amount.
- R is the periodic interest rate, calculated as: R = Annual Interest Rate/12.
- Principal component = EMI - Interest component
For instance, if you have already paid ₹10,00,000 of a ₹25,00,000 home loan, the principal amount remaining is ₹15,00,000. If the interest rate is 10.5% per annum, the monthly interest rate is 0.00875. So, for the first month, the interest component = ₹15,00,000 x 0.00875 = ₹13,125, and the principal component = ₹13,493 - ₹13,125 = ₹368.
You can also use Excel to calculate the principal and interest components of an EMI. Here are the formulas:
- Principal component = PPMT(I, x, n, -p)
- Interest component = IPMT(I, x, n, -p)
- EMI = PMT(I, n, -p)
- I is the monthly interest rate.
- X is the month for which you want to calculate the principal and interest components.
- N is the loan tenure in months.
- P is the principal amount.
Using the same example as before, if you want to calculate the principal and interest components for the 10th month of your loan, you would use the following formulas:
- Principal component = PPMT(0.00875, 10, 120, -1500000) = ₹1106
- Interest component = IPMT(0.00875, 10, 120, -1500000) = ₹9905
- EMI = PMT(0.00875, 10, -1500000) = ₹13,493
So, for the 10th month of your loan, you would pay ₹9,905 in interest and ₹1,106 towards the principal amount.
It is important to understand the interest and principal components of your EMI to efficiently manage your finances and make informed decisions about your loan.
Cash Reserves: Smart Investment Strategy or Missed Opportunities?
You may want to see also

How to calculate the remaining principal balance
To calculate the remaining principal balance on an investment, you will need to know the original principal amount, the interest rate, the length of the investment, and how long you have been paying into it.
The principal is the original sum of money that is borrowed or invested, and it is used as the foundation for calculating interest. There are two types of principal balances: the initial principal, which is the original amount borrowed and used to calculate interest and determine the repayment schedule; and the outstanding principal, which is the remaining balance that continues to accrue interest until the loan is paid off.
A simple interest calculation can be used to determine the remaining principal balance. The formula for this is:
Final Investment Value (A) = Principal Amount (P) + Interest (I)
Interest (I) = Principal Amount (P) x Interest Rate (R) x Time (T)
So, if you know the final investment value, you can rearrange the formula to find the remaining principal balance:
Principal Amount (P) = Final Investment Value (A) - Interest (I)
For example, if you have a final investment value of $26,800, an original loan amount of $22,000, and a term of 4 years, the calculation would be as follows:
Principal Amount (P) = $26,800 - ($22,000 x 0.05 x 4)
Principal Amount (P) = $26,800 - $44,000
Principal Amount (P) = $2,800
This means that the remaining principal balance is $2,800.
It is important to note that simple interest is different from compound interest, where interest is added back into the balance, and each time period includes interest on the interest from the previous period.
Investing Cash: Strategies for Smart Money Allocation
You may want to see also

How to calculate the principal and interest on a simple interest loan
To calculate the principal and interest on a simple interest loan, you'll need to know the principal loan amount, the interest rate, and the loan term in years. The formula for calculating the interest on a simple interest loan is:
Principal loan amount x Interest rate x Loan term in years = Interest
For example, if you take out a five-year loan for $20,000 with an interest rate of 5%, the interest would be $20,000 x 0.05 x 5 = $5,000.
The formula for calculating the principal and interest on a simple interest loan is:
SI = P x R x T
Where:
- SI = Total accrued amount (principal + interest)
- P = Principal amount
- R = Rate of interest per year as a percentage
- T = Time or the number of years of the loan
To calculate the principal and interest separately, you can use the formula:
I = Prt
Where:
- I = Interest
- P = Principal
- R = Interest rate as a decimal
- T = Time period
You can then add the interest to the original principal amount to get the total interest plus principal.
It's important to note that simple interest is different from compound interest. With compound interest, the interest that accumulates is added back into the balance of the loan or investment principal, resulting in sub-calculations for each time period. In contrast, simple interest calculations do not involve adding interest back into the principal balance for subsequent interest calculations.
Additionally, the calculation for the principal and interest on a loan can become more complex if the loan uses a different interest calculation method, such as amortization (commonly used for mortgages) or compound interest (often used for credit cards). In these cases, the interest payments may vary over the course of the loan, and a loan calculator can be helpful in determining the interest and principal amounts.
Robo-Investing: BlueCrest's Smart Strategy for Financial Success
You may want to see also

How to calculate the principal and interest on an amortised loan
To calculate the principal and interest on an amortised loan, you need to understand the difference between simple interest and amortised interest.
Simple Interest
Simple interest is calculated on the original principal amount. The formula for calculating simple interest is:
> Principal loan amount x Interest rate x Loan term in years = Interest
For example, if you take out a five-year loan for $20,000 with a 5% interest rate, the simple interest formula would be $20,000 x 0.05 x 5 = $5,000 in interest.
Amortising Interest
Amortising interest is more complex. With an amortising loan, the part of your payment that goes towards interest decreases over time, while the part that goes towards the principal balance increases. This is usually calculated using an amortisation schedule, which details how much will go towards each component of your loan payment (principal or interest) at various times throughout the loan term.
The formula for calculating the interest on an amortised loan is:
> (Interest rate / Number of payments per year) x Remaining loan balance = Interest paid that period
For example, if you have a $5,000 loan balance and a 6% interest rate that you pay off in monthly instalments, you would divide 0.06 by 12 to get 0.005. Multiplying that number by your remaining loan balance, you find that you will pay $25 in interest in the first month.
To calculate the principal paid in that first month, subtract the interest from your fixed monthly payment. If your monthly payment is $430.33, you will pay $405.33 towards the principal in the first month.
Repeat this process for each month of your loan, using the new remaining loan balance each time. To get the total interest, add all the interest payments together.
Corporations' Cash Investment Strategies: Unlocking Business Growth
You may want to see also
Frequently asked questions
The principal amount is the original sum of money that is borrowed in a loan or placed into an investment.
To calculate the principal amount, you can use the following formula: Principal Amount = EMI (Equated Monthly Instalment) - Interest Amount.
You can use a loan balance calculator that takes into account the original loan terms, the month and year of loan origination, and the number of months that have passed. By adjusting the monthly payment amounts to the actual payments made, you can find the current balance.
To calculate the balance of an investment with simple interest, you can use the formula: A = P(1 + rt), where A is the total accrued amount (principal + interest), P is the principal amount, r is the interest rate as a decimal, and t is the time period.







