Beta is a measure of a stock's volatility in relation to the overall market. It is used to gauge the relative riskiness of a stock and is an integral part of the Capital Asset Pricing Model (CAPM). The market, such as the S&P 500 Index, has a beta of 1.0, and individual stocks are ranked according to how much they deviate from the market. A stock with a beta greater than 1.0 is more volatile than the market, while a stock with a beta less than 1.0 is less volatile. Beta can be calculated using regression analysis, comparing the covariance of the return of the security and the return of the market with the variance of the market returns. While beta can be a useful tool for investors, it has limitations and should be considered alongside other factors when making investment decisions.
| Characteristics | Values |
|---|---|
| Purpose | To determine the risk-return profile of an investment |
| Type of Data | Measure of past performance |
| Data Used | Covariance of the asset's return and the market's return, variance of the market |
| Calculation | Covariance / Variance |
| Interpretation | A high beta represents greater risk for the investor |
| Use | To build a balanced portfolio |
| Limitations | Does not guarantee future returns, less effective over long periods |
What You'll Learn

Calculating 3-year beta
Calculating a 3-year beta involves determining the sensitivity of a stock's price to an index or benchmark, typically the S&P 500. This calculation provides investors with an estimate of the risk associated with a particular stock and its potential returns.
To calculate the 3-year beta, you will need historical data on the stock's prices and the index's prices over the same period. Here is a step-by-step guide on how to calculate a 3-year beta:
Step 1: Collect Historical Data
Ensure you have daily price data for both the stock and the index (S&P 500) for the last three years. You can obtain this data from financial websites like Yahoo Finance or Google Finance.
Step 2: Calculate Returns
Calculate the daily returns for both the stock and the index. The return can be calculated by taking the difference between the current day's closing price and the previous day's closing price, then dividing it by the previous day's closing price.
Step 3: Calculate Covariance
Determine the covariance between the stock's returns and the index's returns. Covariance measures how the returns of the stock and the index move together. You can calculate covariance using the following formula:
> Covariance = ((Sum of (Stock Return - Stock Average Return) * (Index Return - Index Average Return)) / Number of Days)
Step 4: Calculate Variance
Calculate the variance of the index's returns, which measures the volatility of the index. You can calculate the variance using the following formula:
> Variance = ((Sum of (Index Return - Index Average Return)^2) / Number of Days)
Step 5: Calculate Beta
Finally, calculate the 3-year beta by dividing the covariance from Step 3 by the variance from Step 4. This will give you the beta value for the stock over the three-year period.
> Beta = Covariance / Variance
Example:
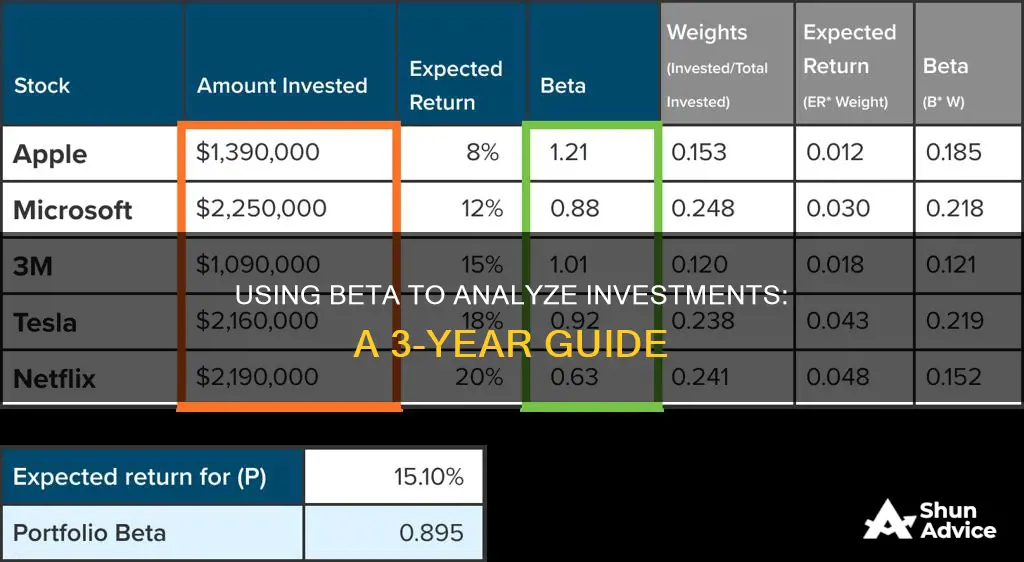
As an example, let's calculate the 3-year beta for Apple (AAPL) stock using data from April 9, 2012, to April 9, 2015.
Assuming we have the daily price data for both Apple and the S&P 500, we can calculate the daily returns for each. Then, we calculate the covariance and variance using the formulas provided. Finally, we divide the covariance by the variance to get the 3-year beta for Apple stock.
By following these steps, you can calculate the 3-year beta for any stock, which will help you analyze the risk and potential returns associated with that investment.
Understanding Investment Cash Flows: A Timeline Perspective
You may want to see also

Interpreting the results
- Beta Coefficient Interpretation: The beta coefficient, calculated as the covariance between stock returns and market returns divided by the variance of market returns, provides insights into the volatility and risk of an investment. A beta of 1 indicates that the investment is equally volatile as the market, while a beta greater than 1 suggests higher volatility and risk, and a beta less than 1 indicates lower volatility and risk.
- Volatility and Risk Analysis: By comparing the beta value of an investment to the benchmark (usually the S&P 500 with a beta of 1), investors can gauge the relative volatility and risk. A higher beta indicates greater price fluctuations, while a lower beta suggests more stable returns.
- Investment Portfolio Considerations: The 3-year beta analysis helps investors construct well-balanced portfolios. For example, including low-beta stocks can provide downside protection during adverse market conditions, while high-beta stocks offer the potential for higher returns but with increased risk.
- Limitations and Cautions: It's important to remember that beta is based on historical data and may not accurately predict future performance. Additionally, beta does not consider the fundamentals of a company, such as earnings and growth potential. Other factors, such as industry-specific risks and economic conditions, should also be considered when interpreting beta results.
- Risk Tolerance and Investment Goals: Interpreting beta results should be aligned with an investor's risk tolerance and investment goals. For risk-averse investors, lower-beta investments may be preferable, while those seeking higher returns may be willing to accept the higher risk associated with higher-beta investments.
- Time Horizon and Beta Dynamics: Beta values can change over time, and short-term fluctuations may differ from long-term trends. Thus, investors should consider the time horizon of their investments when interpreting beta results.
By understanding the implications of a 3-year beta analysis, investors can make more informed decisions about their investment strategies and portfolio construction, balancing risk and return potential to align with their financial objectives.
Investment Strategies: Cash Flow-Agnostic Criteria
You may want to see also

Using beta to gauge risk
Beta is a measure of a stock's volatility in relation to the overall market. It is a statistical measure that compares a stock or portfolio's volatility or systematic risk to a benchmark, usually the S&P 500, which has a beta of 1.0. Beta is calculated by dividing the covariance of the security's returns and the market's returns by the variance of the market's returns over a specified period.
Beta is interpreted as follows:
- Beta = 1: Stock is as volatile as the market
- Beta > 1: Stock is more volatile than the market
- Beta < 1: Stock is less volatile than the market
- Beta = 0: Stock is uncorrelated to the market
- Beta < 0: Stock is negatively correlated to the market
A stock with a beta of 1.0 means its price activity correlates with the market. Adding such a stock to a portfolio doesn't increase the potential for greater returns but also doesn't add much risk. A beta greater than 1.0 indicates that the stock is theoretically more volatile than the market and may lead to higher returns but also increases the portfolio's risk. Conversely, a beta less than 1.0 indicates that the stock is less volatile than the market and will make the portfolio less risky.
Beta is a useful measure for investors to gauge the risk of a stock and how it will impact their portfolio. However, it is more useful for short-term rather than long-term risk analysis as a stock's volatility can change over time. It also doesn't provide information about the fundamentals of a company and doesn't predict future moves.
Investing Before Elections: Cash or Risk?
You may want to see also

Using beta to build a balanced portfolio
Beta is a measure of a stock's volatility in relation to the overall market. The market, such as the S&P 500, has a beta of 1.0, and individual stocks are ranked according to how much they deviate from the market. A stock that swings more than the market over time has a beta above 1.0, whereas a stock that moves less than the market has a beta of less than 1.0. Beta is calculated by dividing the covariance of the security's returns and the market's returns by the variance of the market's returns over a specified period.
When building a balanced portfolio, investors should consider both portfolio balance and beta-weighting. Portfolio balance involves diversifying investments across different securities, option strategies, and multiple contracts to spread out risk. Beta-weighting, on the other hand, helps investors understand how "bullish" or "bearish" their portfolio is relative to a benchmark index. By calculating the beta-weighted portfolio, investors can determine the overall profitability of their portfolio based on the benchmark index's performance.
To calculate the beta-weighted portfolio, investors can use tools such as Microsoft Excel or specific platforms like Think or Swim and Rose Broker. These platforms allow investors to input their portfolio details and the benchmark index they want to track, such as the S&P 500 or SPY. The platform will then provide a payoff distribution curve, showing the potential profit or loss of the overall portfolio at different price points of the benchmark index.
By regularly analysing the beta-weighted portfolio, investors can make more informed decisions about their investment strategies. For example, if the portfolio is currently "bearish", indicating that it will make the most money if the market goes down, investors may consider adding more "bullish" positions to balance their portfolio. This involves searching for and adding investments that will profit if the market moves up. Conversely, if the portfolio is "bullish", investors may consider adding more "bearish" positions to protect against potential losses if the market declines.
In conclusion, by utilising both portfolio balance and beta-weighting, investors can construct a more balanced portfolio that is less susceptible to market direction. Regular analysis of the beta-weighted portfolio helps investors adjust their investment strategies and make more informed decisions about adding or removing positions to maintain a neutral portfolio that is not overly "bullish" or "bearish".
A Beginner's Guide to Investing with Zerodha
You may want to see also

Beta's limitations and alternatives
Beta's Limitations
Beta has several limitations as a measure of risk and volatility. Firstly, it is calculated using historical data points and is therefore less meaningful for predicting a stock's future movements, particularly for long-term investments. A stock's volatility can change significantly over time, depending on various factors such as the company's growth stage. Beta also assumes that stock returns are normally distributed, which is not always the case in reality. Thus, beta's predictions about a stock's future movements may not always be accurate.
Additionally, beta only considers a stock's past performance relative to a benchmark index like the S&P 500 and does not take into account the fundamentals of a company, such as its earnings and growth potential. Beta also fails to distinguish between upside and downside price movements, which is important for investors as downside movements are generally seen as risky, while upside movements present opportunities. Beta also does not account for the price paid for a stock relative to fundamental factors, such as changes in company leadership or future cash flows.
Furthermore, beta is based solely on past price movements, which may not be indicative of future trends. Beta values for individual stocks also tend to fluctuate over time, making them unreliable for long-term predictions. Beta is generally considered more useful for short-term traders than for investors with long-term horizons.
Alternatives to Beta
There are several alternatives to beta that can be used to evaluate investments:
- Sharpe Ratio: Measures the risk-adjusted return of an investment or portfolio by comparing the excess return over the risk-free rate to its volatility (standard deviation of returns). A higher ratio indicates better risk-adjusted performance.
- Sortino Ratio: Similar to the Sharpe ratio, but it only considers the standard deviation of negative returns, focusing on downside risk.
- Treynor Ratio: Evaluates the risk-adjusted performance of an investment relative to its systematic risk (beta). It is calculated by dividing the excess return of the investment over the risk-free rate by its beta. A higher Treynor ratio indicates better risk-adjusted performance relative to market risk.
- Jensen's Alpha: Measures the excess return of an investment relative to its expected return based on its beta and the risk-free rate. It is used to assess whether a portfolio manager has added value through active management after adjusting for market risk.
- R-squared: Measures the percentage of a fund's movements that can be explained by movements in its benchmark index, providing insight into how closely the fund's performance tracks the benchmark. A higher R-squared indicates a stronger correlation.
- Tracking Error: Measures the standard deviation of the difference between the returns of a portfolio or fund and its benchmark index, quantifying how closely the portfolio's returns track the benchmark's returns. A lower tracking error indicates better alignment.
Corporations' Cash Investment Strategies: Unlocking Business Growth
You may want to see also
Frequently asked questions
Beta is a measure of an investment's volatility or risk compared to the overall market, typically over three years. It indicates how much an asset's returns fluctuate relative to a benchmark, usually the S&P 500.
The beta coefficient is calculated by dividing the covariance of the asset's return and the market's return by the variance of the market's return over three years. This helps determine how sensitive an asset's returns are to market movements.
A 3-year beta value greater than 1 suggests that the investment is more volatile than the market. This means the investment's returns are expected to fluctuate more than the benchmark index.
A 3-year beta can help investors assess the risk and potential returns of an investment. It provides insight into how sensitive an investment is to market changes and can help build a balanced portfolio that aligns with an investor's risk tolerance.







