When it comes to investing, it's important to understand the concept of compound interest, which is the interest you earn not only on your original investment but also on the interest that accumulates over time. This means that your savings or investments can grow at an increasing rate. To calculate compound interest with monthly contributions, you can use the formula A=P(1+r/n)^nt, where:
- A = ending amount
- P = original investment amount
- r = annual interest rate (as a decimal)
- n = number of times interest is compounded per year
- t = number of years
This formula assumes that interest is calculated before the regular monthly contribution is added. It's important to note that different calculators or methods may yield slightly different results, so it's always a good idea to double-check your calculations and ensure you're using the correct formula for your specific situation.
| Characteristics | Values |
|---|---|
| Principal Investment | P |
| Monthly Contributions | PMT |
| Annual Interest Rate | r |
| Compounds per Year | n |
| Total Contributions | P + PMT*n |
| Interest on Principal | Prt |
| Interest on Contributions | PMT*[(1+r)^n-1]/r-PMT |
| Future Value of Investment | P(1+r)^nt + PMT* [((1+r)^nt - 1)/r] |
What You'll Learn

Calculating compound interest
The Compound Interest Formula
The formula for calculating compound interest is:
> A = the future value of the investment
> P = the principal balance
> r = the annual interest rate (as a decimal)
> n = number of times interest is compounded per year
> t = the time in years
> ^ = ... to the power of ...
This formula can be used to calculate compound interest for different periods, such as monthly or annually, by adjusting the 'n' value. For example, for monthly compounding, 'n' would be 12, and for annual compounding, 'n' would be 1.
Examples of Compound Interest Calculations
- Monthly compounding: To calculate monthly compound interest, divide the annual interest rate by 12 (the number of months in a year), add 1, and raise this result to the power of 12 t (years).
- Annual compounding: For annual compounding, you can use the formula A=P(1+r/n)^(nt). For example, if you deposit $5,000 in a savings account with a 5% annual interest rate, compounded monthly, you would calculate A = $5,000(1 + 0.00416667/12)^(12 x 1), resulting in an ending balance of $5,255.81 after one year.
Basic Investment Formula
A basic investment formula assumes that you start with an initial principal amount, invest it at an annually compounded rate of return, and add equal contributions every year. The formula for this is:
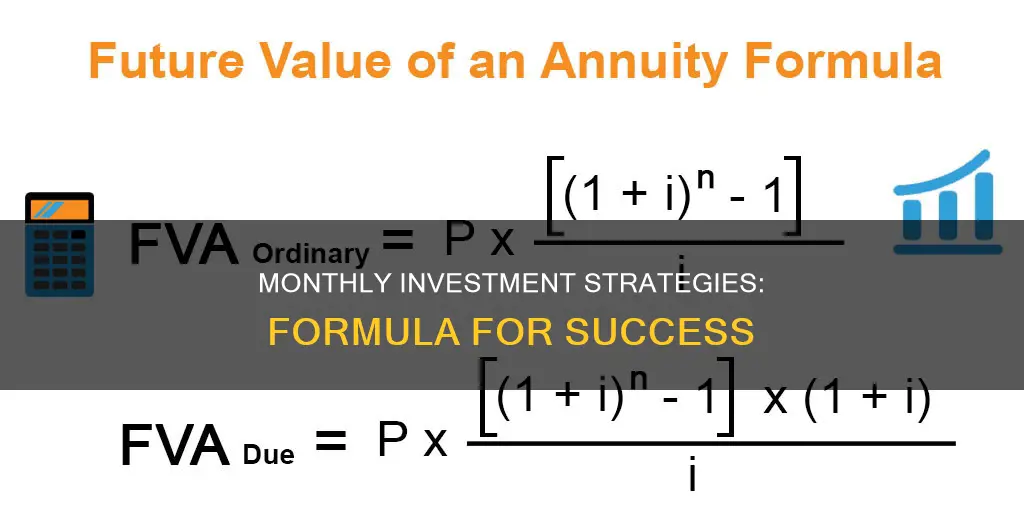
Balance(Y) = P(1 + r)Y + c[ ((1 + r)Y + 1 - (1 + r)) / r ]
This formula assumes contributions occur at the start of each year. If you want them to occur at the end of the year, the formula becomes:
Balance(Y) = P(1 + r)Y + c[ ((1 + r)Y - 1) / r ]
Compound Interest with Regular Monthly Contributions
> FV= P(1 +r/n) ^ (nt) + PMT * [(((1 + r/n) ^ (nt)) -1 ) / (r/n)]
Where:
> FV = Future Value
> P = Principal
> r = returns per annum
> n = compound monthly
> PMT = monthly contribution amount
> t = number of investment years
Strategies for Making the Most of Compound Interest
- Start Early: Time is a crucial factor in compound interest. Starting investments early, even with smaller amounts, allows more time for your money to grow and for interest to compound.
- Regular Contributions: Consistently adding to your investment enhances the compounding effect. Each new contribution earns its own interest, boosting the overall growth.
- Higher Compounding Frequencies: When choosing savings or investment options, consider those that compound more frequently. More frequent compounding can lead to greater growth potential, although the difference may be minimal at lower interest rates.
Understanding Cash Flows from Ordinary Investing Activities
You may want to see also

Annual yield on savings
When it comes to annual yield on savings, there are a few key concepts and formulas to consider.
First, it's important to understand the difference between Annual Percentage Yield (APY) and Annual Percentage Rate (APR). APY is the rate of return you can expect to earn on your investment in a given year, including compound interest. APR, on the other hand, is used for loans and reflects the effective percentage that a borrower will pay over a year in interest and fees. APY is particularly useful for comparing different investment options and financial institutions to ensure you're getting the highest possible return.
The formula for calculating APY is:
APY = (1 + r/n)^n - 1
Where:
- R = period rate
- N = number of compounding periods
For example, if you deposit $100 for one year at a 5% interest rate compounded quarterly, you would calculate APY as follows:
APY = (1 + 0.05/4)^4 - 1 = 0.05095 = 5.095%
So, at the end of the year, your $100 would have grown to $105.09.
Now, let's consider the impact of regular contributions. Using the compound interest formula:
A = P(1 + r/n)^nt
Where:
- A = ending amount
- P = original balance
- R = interest rate (as a decimal)
- N = number of times interest is compounded in a specific time frame
- T = time frame
For example, if you deposit $5,000 in a savings account with a 5% annual interest rate compounded monthly, you would calculate the ending amount as follows:
A = $5,000(1 + 0.00416667/12)^(12 x 1) = $5,255.81
So, after one year, you would have $5,255.81 in savings.
Let's say you also contribute an additional $100 at the end of each month. Using the same formula, you would calculate the ending amount as follows:
A = $5,000(1 + 0.00416667/12)^(12 x 10) + $100(1 + 0.00416667/12)^(11 x 10) + ... + $100(1 + 0.00416667/12)^(1 x 10) = $15,255.81
So, after one year, you would have a total of $15,255.81 in savings, including your contributions.
It's worth noting that savings accounts are not the only way to save and earn passive income. There are alternative investments, such as Certificates of Deposit (CDs) and Treasury bills, that can offer higher returns. Additionally, the inflation rate in the US is typically higher than savings account returns, so it's important to consider other investment options to maximize your returns and preserve your purchasing power.
Cashing Out Investments: Using the Cash App to Withdraw Funds
You may want to see also

Monthly contribution amount
The monthly contribution amount is a key component of investment plans, and understanding the relevant formulas can help individuals make informed decisions about their financial strategies.
The monthly contribution amount, often denoted as "PMT" or "c", represents the regular payment made towards an investment or savings account. This amount is added to the initial principal or investment balance. The formula for calculating the future value of an investment with monthly contributions is:
> FV = P(1 + r/n) ^ (nt) + PMT * [(((1 + r/n) ^ (nt)) - 1) / (r/n)]
Here, "FV" is the future value of the investment, "P" is the initial principal, "r" is the annual interest rate, "n" is the number of compounding periods per year, and "t" is the number of years.
For example, let's consider an investment with an initial principal of $1,000, an annual interest rate of 5% (or 0.05 as a decimal), compounded monthly (so n = 12), and a monthly contribution of $100 for 10 years (t = 10).
Plugging these values into the formula:
> FV = 1000(1 + 0.05/12) ^ (12 * 10) + 100 * [(((1 + 0.05/12) ^ (12 * 10)) - 1) / (0.05/12)]
Calculating this equation will give the future value of the investment, including the impact of the monthly contributions.
It's important to note that the timing of contributions can vary, and this will impact the final balance. Some investments assume contributions are made at the start of each period, while others assume contributions are made at the end. This should be considered when using formulas to ensure an accurate representation of the investment's performance.
Cash App Investing: Free or Fee-Based?
You may want to see also

Initial investment amount
When it comes to investing, there are several factors to consider, including the initial investment amount, also known as the principal or starting amount. This is the amount you have at the beginning of the investment period.
The initial investment amount is an important figure because it serves as the foundation for your investment portfolio. It is the amount you are willing and able to commit, which will then be supplemented by any additional contributions you make over time. This starting sum can be derived from various sources, such as savings, bonuses, gifts, or inheritances.
When determining the initial investment amount, it is crucial to assess your financial situation and goals. This amount should be significant enough to serve as a solid base for your investment strategy, but it should also be manageable and within your means. It is generally advisable to invest only what you can afford to lose, especially when dealing with riskier investments.
Additionally, the initial investment amount can vary depending on the type of investment and the requirements of the brokerage firm or financial institution. For instance, some mutual funds and index funds may have minimum starting balance requirements, typically ranging from a few hundred dollars to $1,000 or more. On the other hand, individual equities and bonds usually have no minimum investment requirements, allowing you to start with a smaller initial investment amount.
In conclusion, the initial investment amount is a critical component of your overall investment strategy. It sets the tone for your financial commitment and should be carefully considered in relation to your risk tolerance, investment goals, and financial capabilities.
Deferred Revenue Cash: Invest or Not?
You may want to see also

Total value of investment
The total value of an investment with monthly contributions can be calculated using the compound interest formula. Compound interest is the interest earned on the original investment, as well as the interest accumulated on that interest.
The formula for calculating the total value of an investment with monthly contributions is:
FV= P(1 + r/n) ^ (nt) + PMT * [(((1 + r/n) ^ (nt)) -1 ) / (r/n)]
Where:
- FV = Future Value (total value of the investment at the end of the period)
- P = Principal (the initial investment amount)
- R = Annual interest rate (as a decimal)
- N = Compound frequency (number of times interest is compounded per year)
- T = Time (number of years the investment is held for)
- PMT = Monthly contribution amount
For example, if you invest $1,000 with an annual interest rate of 5% (0.05 as a decimal), compounded monthly (n=12), and you contribute an additional $100 at the end of each month, the formula would be:
FV = 1000(1 + 0.05/12)^(12 x 5) + 100 * [(((1 + 0.05/12)^(12 x 5)) -1 ) / (0.05/12)]
This calculates the future value of the investment after 5 years, taking into account both the initial investment and the monthly contributions.
The formula can be adjusted to account for contributions made at the start of each month, or to calculate the value of the investment at any given time by changing the value of 't'.
Fidelity Investments and Zelle: What's the Connection?
You may want to see also
Frequently asked questions
The formula for calculating compound interest with monthly contributions is:
A=P(1+r/n)^(nt)
Where:
- A = ending amount
- P = original balance
- r = interest rate (as a decimal)
- n = number of times interest is compounded in a specific time frame
- t = time frame
The formula for calculating compound interest is the same as above:
A=P(1+r/n)^(nt)
To calculate the ending amount, you will need to know the original balance, the interest rate, the number of times the interest is compounded in the given time frame, and the time frame itself. Plug these values into the formula above and calculate the result.
To calculate compound interest with regular monthly contributions, you can use the following formula:
FV= P(1 +r/n) ^ (nt) + PMT * [(((1 + r/n) ^ (nt)) -1 ) / (r/n)]
Where:
- FV = Future Value
- P = Principal Investment (initial deposit)
- PMT = Monthly Contribution
- r = Annual Interest Rate
- n = Compounds per year
- t = Number of years
To calculate the future value of your investment, you can use the formula provided in the previous answer. Ensure that you have accurate values for all the variables in the formula, and then calculate the result.







