Unraveling The Power Of Compound Interest: A Math Journey To Investment Success

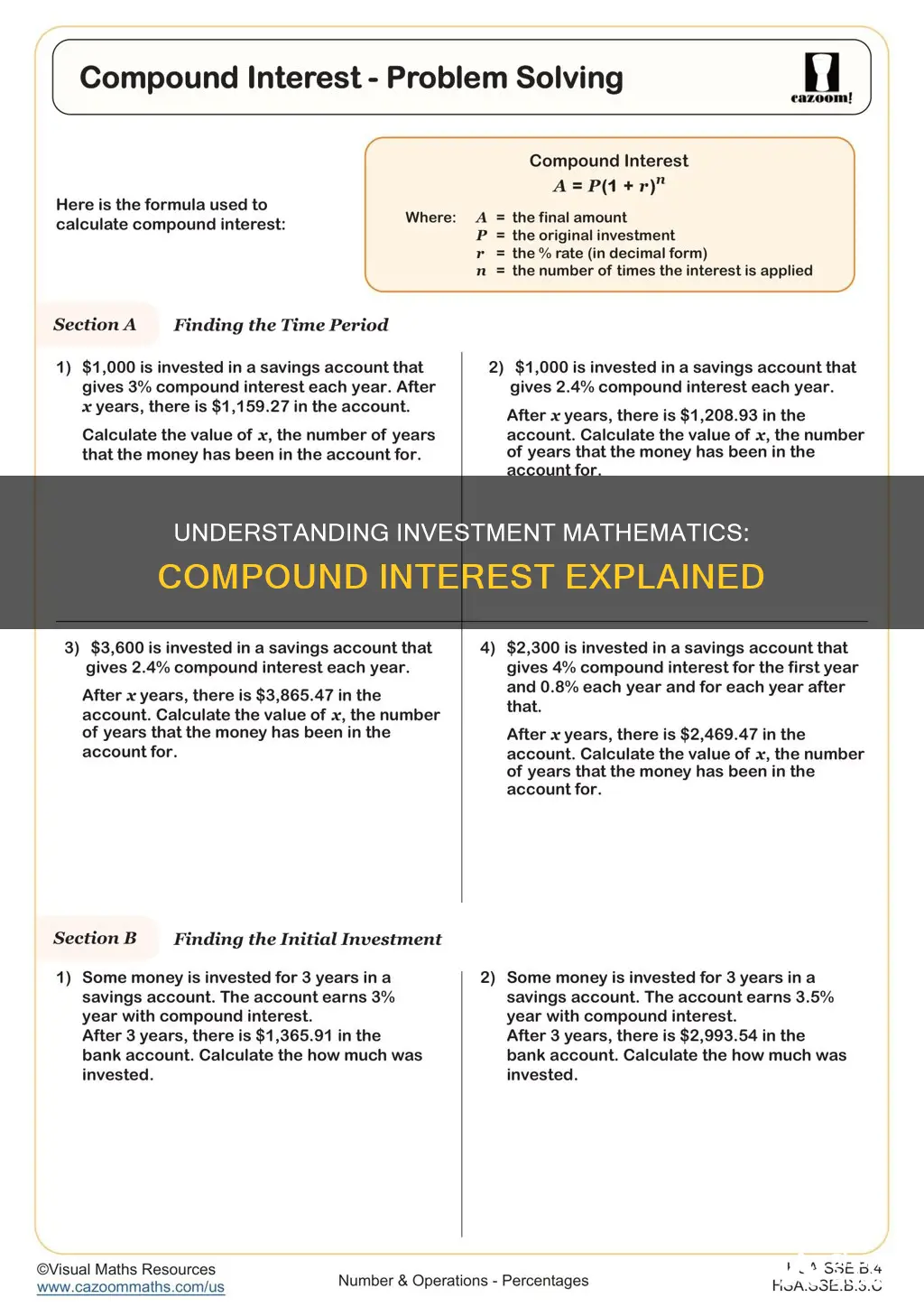
Compound interest is a fundamental concept in mathematics and finance, representing the process by which an initial amount of money grows exponentially over time. It occurs when interest is added to the principal amount, and the subsequent interest is then earned on the new total. This concept is a powerful tool in investment strategies, as it allows for the potential of significant returns over extended periods. Understanding compound interest is crucial for investors and savers, as it can help them make informed decisions about their financial goals and the growth of their investments.

Compound Interest Formula: A simple equation to calculate future value

Compound interest is a powerful concept in finance, allowing investments to grow exponentially over time. It is the interest calculated on the initial principal amount, which also includes the accumulated interest from previous periods. This concept is a fundamental principle in investment, as it enables investors to earn interest on their earnings, leading to significant growth over extended periods. Understanding the formula for compound interest is essential for anyone looking to make informed financial decisions.

The formula for calculating the future value of an investment with compound interest is straightforward and can be expressed as:

FV = P(1 + r/n)^(nt)

Where:

• FV represents the future value of the investment.
• P is the principal amount (the initial amount of money).
• R is the annual interest rate (expressed as a decimal).
• N is the number of times interest is compounded per year.
• T is the time the money is invested for, in years.

This formula provides a clear method to determine the total value of an investment at a future date. It takes into account the regular addition of interest, which is a key feature of compound interest. The more frequently interest is compounded, the more significant the impact on the final amount. For instance, if interest is compounded annually, the formula simplifies to FV = P(1 + r)^t, making it easier to calculate.

To illustrate, let's consider an example. Suppose you invest $5,000 at an annual interest rate of 5%, compounded annually. Using the formula, you can calculate the future value after 10 years. Plugging in the values, you get FV = $5000 * (1 + 0.05)^10, which results in a future value of approximately $7,786. This demonstrates how compound interest can significantly increase the value of your investment over time.

Mastering this formula is crucial for investors and individuals planning for long-term financial goals. It empowers them to make accurate projections and understand the potential growth of their investments. By applying the compound interest formula, one can make informed decisions regarding savings, retirement planning, or any investment strategy, ensuring a more secure financial future.

Compounding Periods: Interest applied at regular intervals

Compound interest is a fundamental concept in finance, representing the growth of an investment or debt through the addition of interest to the principal amount. It is a powerful tool that can significantly impact the value of money over time. When interest is applied at regular intervals, it is known as a compounding period, and this process can lead to exponential growth or savings.

In the context of investments, compounding periods refer to the frequency at which interest is calculated and added to the principal. For example, if you invest $1000 at an annual interest rate of 5%, the interest for the first year would be $50, making the total $1050. In the second year, the interest is calculated based on the new total of $1050, resulting in $52.50 of interest earned, and so on. This process repeats for each compounding period, whether it's annually, semi-annually, quarterly, monthly, or even daily.

The key to understanding compounding periods is recognizing that the interest is not just earned on the initial investment but also on the accumulated interest from previous periods. This creates a snowball effect, where the investment grows faster and faster with each compounding cycle. For instance, in the previous example, after two years, the investment would grow to $1102.50, and after three years, it would be $1157.63, demonstrating the power of compounding.

The frequency of compounding directly influences the final amount. More frequent compounding periods result in a higher total, as the interest is calculated and added more often. For instance, daily compounding will yield a slightly higher return compared to monthly compounding, which, in turn, will be higher than annual compounding. This is because the interest is earned more frequently, allowing for more rapid growth.

Understanding compounding periods is crucial for investors as it allows them to estimate the potential growth of their investments accurately. By knowing the compounding frequency, investors can make informed decisions about their financial strategies, whether it's choosing the right savings account, planning for retirement, or investing in various financial instruments. It empowers individuals to take control of their financial future and make the most of their money.

Continuous Compounding: Interest calculated infinitely often

Continuous compounding is a method of calculating interest that assumes interest is compounded infinitely often over a given period. This concept is a more precise and theoretical approach compared to the discrete compounding methods commonly used in financial calculations. In continuous compounding, the interest is calculated and added to the principal amount at every infinitesimally small time interval, resulting in a smooth and continuous growth curve.

The formula for continuous compounding is derived from the concept of differential calculus and is given by:

A = P * e^(rt)

Where:

• A is the amount of money accumulated after n years, including interest.
• P is the principal amount (the initial amount of money).
• E is the base of the natural logarithm, approximately equal to 2.71828.
• R is the annual interest rate (in decimal).
• T is the time the money is invested for, in years.

In this formula, the interest rate 'r' is treated as a continuous variable, and the time 't' is considered to be infinitely divisible. The exponential function 'e^(rt)' represents the growth factor, which is the factor by which the principal amount is multiplied over time.

Continuous compounding is often used in theoretical discussions and academic settings to illustrate the power of compound interest. It provides a more accurate representation of the concept, especially when dealing with very long investment periods or very small interest rates. For example, if you invest a small amount at a very low interest rate, the difference between continuous and discrete compounding becomes significant over a long period, as the continuous method accounts for the interest being calculated and added at every instant.

This method is particularly useful in understanding the behavior of interest rates in financial markets and the impact of compounding on investment growth. It allows investors and mathematicians to model complex scenarios and make more accurate predictions about the future value of investments. However, in practical financial applications, discrete compounding periods are typically used due to the limitations of calculating and compounding interest infinitely often in real-world scenarios.

Compound Interest Tables: Pre-calculated values for quick reference

Compound interest is a powerful concept in finance, representing the growth of an investment over time, where interest is calculated not only on the initial principal but also on the accumulated interest from previous periods. This means that the longer an investment is left to grow, the more significant the impact of compound interest becomes. It is a fundamental principle in investment, savings, and debt management, and understanding it is crucial for anyone looking to make informed financial decisions.

A compound interest table is a useful tool that provides pre-calculated values for quick reference, making it easier to estimate the future value of an investment or the amount of interest accrued over time. These tables are particularly handy for financial professionals, investors, and individuals who want to plan their financial strategies efficiently. By using these tables, one can quickly determine the impact of different interest rates, compounding periods, and investment durations without having to perform complex calculations manually.

The tables typically present a range of values for various investment scenarios, allowing users to compare different options. Each table entry represents a specific combination of principal amount, interest rate, and compounding frequency, along with the corresponding future value or interest accrued. For instance, a table might show how a $1,000 investment grows over 10 years at different interest rates, say 5% and 8%, with monthly and yearly compounding. This information is invaluable for making apples-to-apples comparisons between various investment products or strategies.

Creating compound interest tables involves using the formula for compound interest: A = P(1 + r/n)^(nt), where A is the future value, P is the principal amount, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the time in years. By plugging in different values for these variables, one can generate a comprehensive table covering a wide range of investment scenarios.

In summary, compound interest tables are essential resources for anyone dealing with investments and savings. They provide a quick and efficient way to understand the potential growth of investments and the impact of different interest rates and compounding periods. With these tables, individuals can make more informed decisions about their financial future, whether it's choosing the right savings account, planning for retirement, or deciding on the best investment strategy.

Real-World Applications: Compound interest in savings, loans, and investments

Compound interest is a powerful concept that can significantly impact your financial journey, whether you're saving for the future, borrowing money, or investing. It refers to the process where interest is calculated not only on the initial amount but also on the accumulated interest from previous periods. This means that your money can grow exponentially over time, making it an essential tool for wealth creation and financial planning.

In the context of savings, compound interest works in your favor. When you deposit money into a savings account, it earns interest, and this interest, in turn, earns interest in subsequent periods. The longer your money remains in the account, the more it grows. For example, if you invest $1,000 at an annual interest rate of 5%, after the first year, you'll have $1,050. In the second year, you'll earn interest on the new total, resulting in $1,102.50. This snowball effect can lead to substantial savings over time, especially when combined with regular contributions.

When it comes to loans, compound interest can work against you. This type of interest is often associated with credit cards, personal loans, and mortgages. When you borrow money, you're not only repaying the principal but also the interest that accrues over time. The longer it takes to repay the loan, the more interest you'll pay. For instance, a credit card with a 15% annual interest rate will charge interest on the outstanding balance, leading to a growing debt if not managed properly. Understanding compound interest is crucial for borrowers to estimate the total cost of borrowing and plan their repayment strategies.

Investors can leverage compound interest to maximize returns. Compound interest is a key factor in the success of long-term investment strategies. When you invest in stocks, bonds, or mutual funds, your initial investment grows, and the earnings generate additional earnings over time. This compounding effect can turn modest investments into substantial sums. For instance, investing $5,000 in a stock fund with an annual return of 8% for 20 years could result in a final value of over $20,000, demonstrating the power of compound interest in growing wealth.

In summary, compound interest is a fundamental concept with real-world implications. It can be a powerful ally in building savings, a double-edged sword in borrowing, and a key driver of investment growth. Understanding how it works is essential for making informed financial decisions, ensuring that your money works harder for you and helping you achieve your financial goals, whether it's saving for retirement, paying off debt, or growing your wealth.

Frequently asked questions